

Next: Air-Bladder Inflation System.
Up: Energy Budget for JES
Previous: The Total Energy Budget
The APEX 180ml Buoyancy Engine.
The energy consumed by the high pressure pump motor is given by
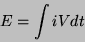 |
(2) |
where i is the electrical current, V is the voltage, and t is time.
For subsequent computations, it will be convenient to express this integral
in terms of the volume pumped rather than time. The following model of the
pump motor applies,
 |
(3) |
where
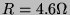 is the internal resistance of the motor,
is the internal resistance of the motor,
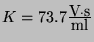 is a generation
factor for back-EMF, and
is a generation
factor for back-EMF, and
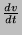 is the volume pump-rate (see
Appendix A). Using (3) to
effect a change-of-variables in (2) yields,
is the volume pump-rate (see
Appendix A). Using (3) to
effect a change-of-variables in (2) yields,
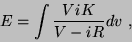 |
(4) |
Due to active ascent-rate control, the energy consumed by the buoyancy
engine occurs at unpredictable depths while measuring the hydrographic
profile. Figure 1 shows a profile of in situ density
for the upper 800 decibars of the JES. A reasonable estimate is obtained by
considering the buoyancy generation to have occurred at only 4 points in the
water column (marked with blue circles in Figure 1).
Accordingly, (2) can be approximated as
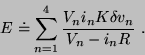 |
(5) |
Figure 1:
This is a plot of the in situ density of the JES.
|
|
- 1.
- The initial piston extension at the parking depth of 800 dbars
increases the buoyancy by 31ml. This buoyancy is considered to be a
marginal force above and beyond that required for neutral buoyancy at any
given depth and its function is to maintain the float's ascent rate. The
energy consumed by pumping 31ml against 800 dbars of pressure is
 |
(6) |
- 2.
- The buoyancy difference between the parking depth and the base of the
pycnocline (
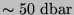 )
is
)
is
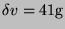 (see
Appendix B). The energy consumed by pumping 44ml against
450 dbar of pressure is
(see
Appendix B). The energy consumed by pumping 44ml against
450 dbar of pressure is
 |
(7) |
- 3.
- The buoyancy difference between the base of the pycnocline (
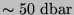 )
and the surface is
)
and the surface is
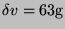 (see
Appendix B). The energy consumed by pumping 66ml against
50 dbar of pressure is
(see
Appendix B). The energy consumed by pumping 66ml against
50 dbar of pressure is
 |
(8) |
- 4.
- This model of APEX has 180ml of gross displacement capacity but
there is only 157ml of displacement available between the ballast piston
position (24 A/D counts) and full piston extension (248 A/D counts).
The displacements in items 1-3 have consumed 138ml leaving 16ml to be
pumped at the surface in order for the piston to reach full extension.
The energy consumed by pumping 16ml against atmospheric pressure is
 |
(9) |
According to (5), the total energy consumed by the APEX buoyancy
engine during each profile is
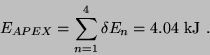 |
(10) |


Next: Air-Bladder Inflation System.
Up: Energy Budget for JES
Previous: The Total Energy Budget
Dana Swift, swift@ocean.washington.edu